Integration is generally thought of as finding the area between two curves, with usually one of the curves being the x-axis itself. The idea of integration, finding the area under the curve, is quite old. It can date back all the way to antiquity to Archimedes himself. Archimedes method is known as his method of quadratures. However, this method is limited in application, being really only applicable to scenarios where the curve itself requires high geometric symmetry. One merely needs to move beyond parabolas to see where difficulties arise with this method of quadratures.

The idea behind this method of quadratures starts with the blue triangle. Then it creates a pair of triangles within the empty space, of which more pairs of empty triangles are placed in the empty space. Archimedes showed that by summing the areas of the triangles in a term by term manner, there exists an infinite geometric series which can then be calculated easily and yield the result of the area in question.

Integration reached an important milestone with the discoveries of Newton and Leibniz, both of whom recognized the intrinsic connection between differentiation and integration. Newton’s method in particular noticed the critical connection when comparing velocity time graphs to distance time graphs. This connection is the Fundamental Theorem of Calculus. Anti-derivatives suddenly became more than a party trick, as they were essential to integration. Finding the area beneath curves suddenly appeared to become limitless, so long as you could find an anti-derivative. It also changed the perception of an integral. Remember that the goal of integration prior to calculus was to find the area underneath a curve. With Calculus’ arrival, this problem seemed to have been reduced to merely finding anti-derivatives.

However, unlike Archimedes’ method, the idea of an integral via Calculus developed in the 17th century was not thought of as rigorous by mathematicians. It was not until the 19th century when Cauchy developed his idea of epsilon-delta statements, and then applied it to integration. The idea of partitioning the area under the curve infinitely is essentially what Cauchy arrived at with his claim about what an integral is. Each partition would then be multiplied with a corresponding functional “height” to gain an area of a box. With the partitions, he could make various Cauchy sums based on the number of partitions. He claimed that the integral is essentially the limit of the Cauchy sums as the number of partitions goes to infinity.

However, unlike Cauchy, Dirichlet considered the many possibilities that arose with discontinuity, and he would certainly not be satisfied with merely having an integral defined for everywhere continuous functions.

This leads to Riemann. He thought to ask about the convergence of Cauchy sums. In his approach, he looked at the Cauchy sums that resulted from overestimations and underestimations. As the partitions grew in number, the overestimations would become less of an overestimate and the underestimations would become less of an underestimate. Thus, the value that these sequences of overestimates and underestimates converge to is what we can think of as the integral. Even this method leads to some complications, when considers this particular problem:

The answer appears to be obvious, yet the Rieman integral does not allow for such a calculation. On the interval yet . Thus the convergence is not uniform, and one cannot conclude the intuitive result that the integral would be 0. This leads to a final clarification of the integral with the Lebesgue integral. In all the previous integrals, the partitioning was done over the domain of the function. With Lebesgue integrals, this partitioning is performed on the range of the function.

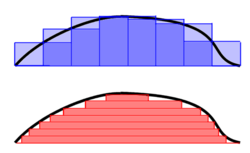
The upper image represents the partitioning within a Riemann Integral,

whereas the lower image demonstrates a Lebesgue Integral

Any Riemann integral must have a Lebesgue integral. Furthermore, every bounded function on a closed interval must have a Lebesgue integral, which leads to the idea that many Lebesgue integrals do not have a Riemann counterpart. Although the Lebesgue integral is not an end-all solution to every problem, it solves many of the problems associated with Riemann integrals and furthermore gives rigor the idea of an integral itself. However, when partitioning the range of the function, it is not clear how much of the domain is in a particular region of partition–thus leads to the need for measuring such a partition. This leads into an entirely different space of mathematics–the theory of measures, where a notion of “measure” to subsets of an Euclidean space is tackled.